
The equation of a circle in general form is, x 2 + y 2 + Dx + Ey + F = 0, where D, E, and F are real numbers. Since any point on the circle is an equal distance, r, from the center. Referencing the figure, we can use the Pythagorean Theorem to find that the equation for this circle in standard form is Given that point (x, y) lies on a circle with radius r centered at the origin of the coordinate plane, it forms a right triangle with sides x and y, and hypotenuse r. We can also derive this equation as follows. We can see this by plugging (0, 0) in for (h, k) in the standard equation of a circle. The standard equation of a circle centered about the origin is x 2 + y 2 = r 2. Standard equation for a circle centered at the origin This is the standard equation of a circle centered about the origin. Notice that if the circle is centered at the origin, (0, 0), then both h and k in the equation above are 0 and the equation reduces to: Substituting the coordinates of the center and radius we get,
Squaring both sides of the equation, yields the standard equation of a circle:įind the equation of the circle with center (4, -3) and radius 5. Given a circle with radius, r, centered at point (h, k), we can use the distance formula to find that The standard equation of a circle is derived using the distance formula. Derivation of the standard equation of a circle This equation can be used for a circle that lies anywhere in the coordinate plane. The standard equation of a circle is (x - h) 2 + (y - k) 2 = r 2. In coordinate geometry, a circle can be expressed using different equations and based on various constraints. Where C is the circumference and π is a mathematical constant approximately equal to 3.14159. Where d is the diameter and π is a mathematical constant approximately equal to 3.14159. Where r is the circle's radius and π is a mathematical constant approximately equal to 3.14159. The figure below depicts the area of a circle in red bounded by the circumference in grey. The area of a circle is the plane region bounded by the circle's circumference.
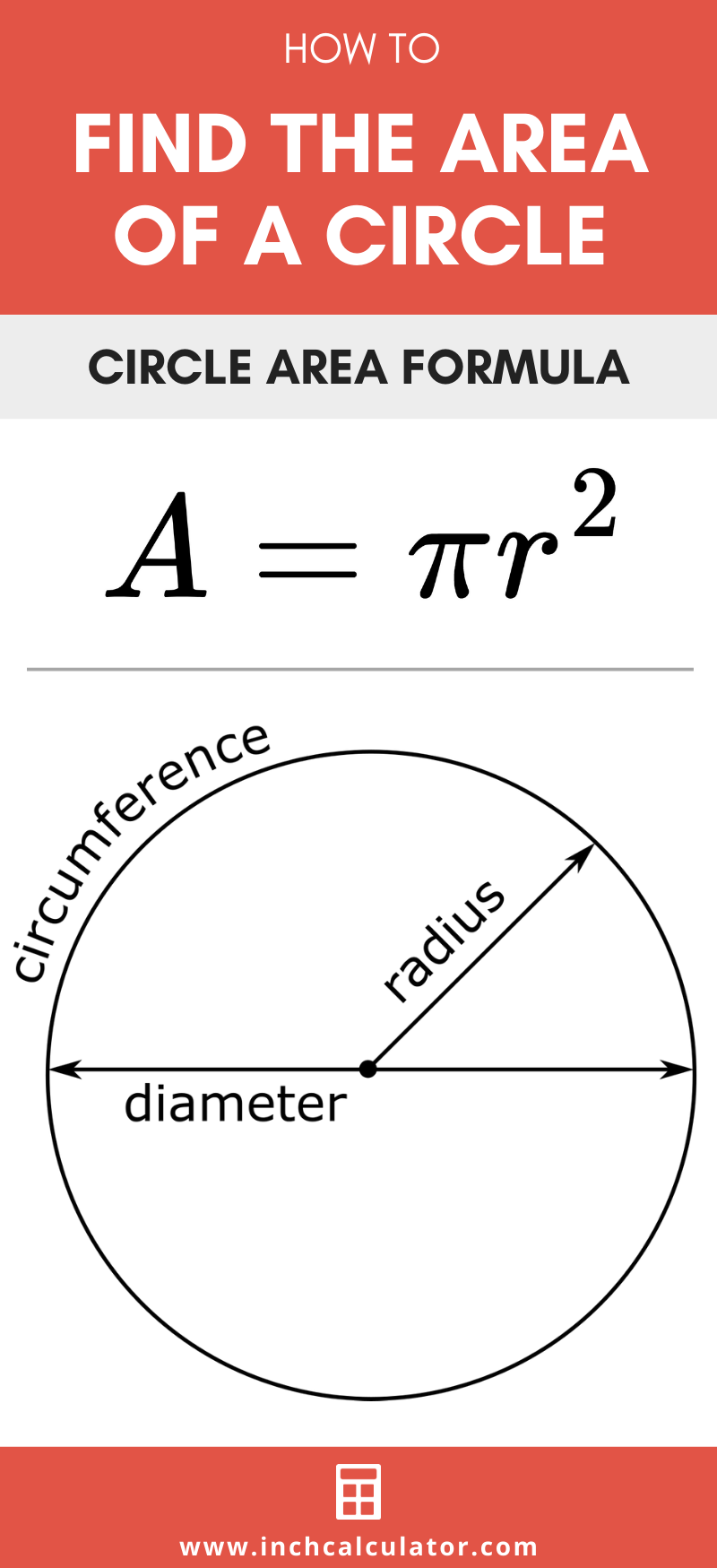
The area of a circle formula is A = πr 2.
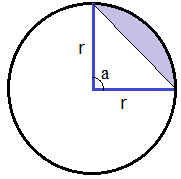
The arc length, s, of a circle is, where θ is the central angle, as shown in the figure below.

Where A is area and π is a mathematical constant approximately equal to 3.14159. There are a few circumference of a circle formulas. Imagine cutting the circle and straightening it out the length of the straightened line is the circumference. Circumference is a measure of the distance around the circle. The circumference of a circle is C = 2πr. The diameter of a circle, is d = 2r, where d is the diameter and r is the radius: The figure below shows the key parts of a circle that we need to know to be able to work with circles and their formulas. There are many circle formulas, such as the area of a circle formula, circumference formula, and diameter formula, all of which are discussed below along with the equations for a circle. Home / geometry / circle / circle formula Circle formulaĪ circle is defined as the set of all points equidistant from a fixed point on a plane.


 0 kommentar(er)
0 kommentar(er)
